91 Inverse functions Informally, two functions f and g are inverses if each reverses, or undoes, the other More precisely Definition 911 Two functions f and g are inverses if for all x in the domain of g , f(g(x)) = x, and for all x in the domain of f, g(f(x)) = x Example 912 f = x3 and g = x1 / 3 are inverses, since (x3)1 / 3 = xPlease Subscribe here, thank you!!!For every function f, subset X of the domain and subset Y of the codomain, X ⊂ f −1 (f(X)) and f(f −1 (Y)) ⊂ Y If f is injective, then X = f −1 (f(X)), and if f is surjective, then f(f −1 (Y)) = Y For every function h X → Y, one can define a surjection H X → h(X) x → h(x) and an injection I h(X

Q 16 Classify The Following Functions F X Defined In Rrarrr As Injective Surjective Both O Youtube
F(x)=x/x^2+1 is bijective
F(x)=x/x^2+1 is bijective- Let the function f R → R be defined by f (x) = cos x, ∀ x ∈ R Show that f is neither oneone nor onto asked in Sets, Relations and Functions by Chandan01 ( 512k points) relations and functions Putting f (x1) = f (x2) we have to prove x1 = x2 Since x1 & x2 are natural numbers, they are always positive Hence, x1 = x2 Hence, it is oneone (injective) Check onto (surjective) f (x) = x2 Let f (x) = y , such that y ∈ N x2 = y x = ±√𝑦 Putting y = 2 x = √2 = 141 Since x is not a natural number Given function f is not onto So, f is not onto (not surjective)



Show That The Function F R X R 1 R 1 Defined By F X X 1 X X R Is One One And Onto Function Hence Find F 1 X
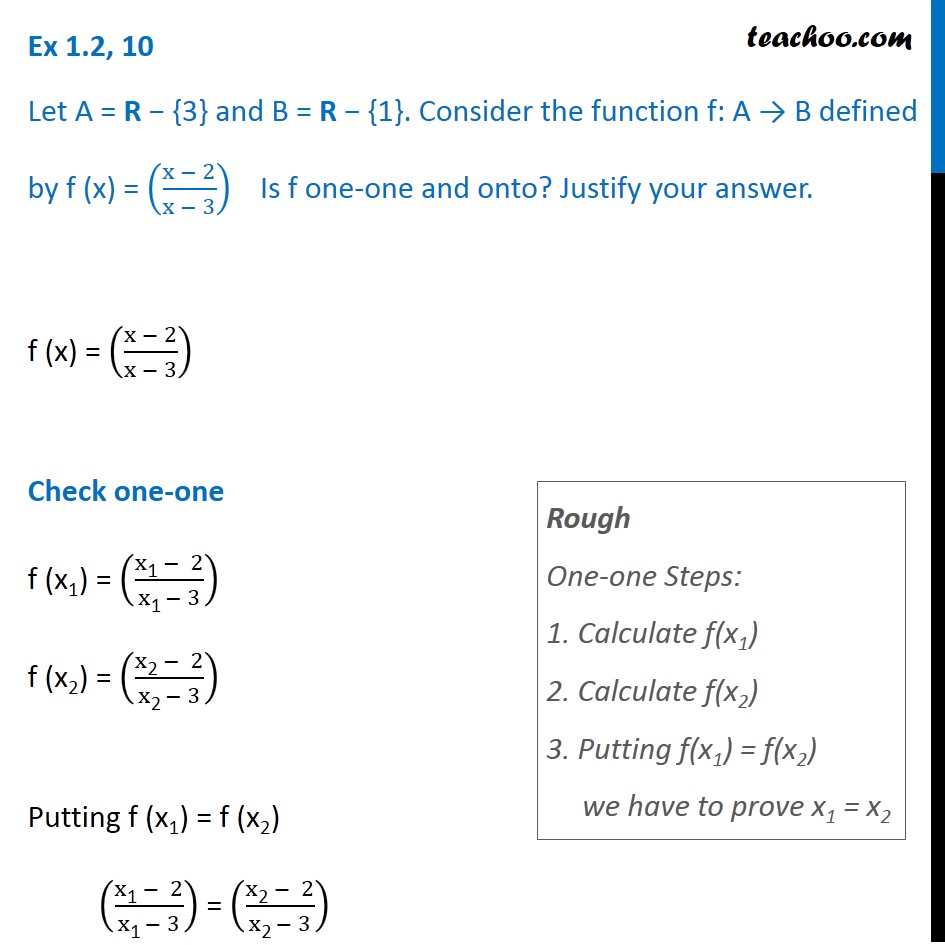
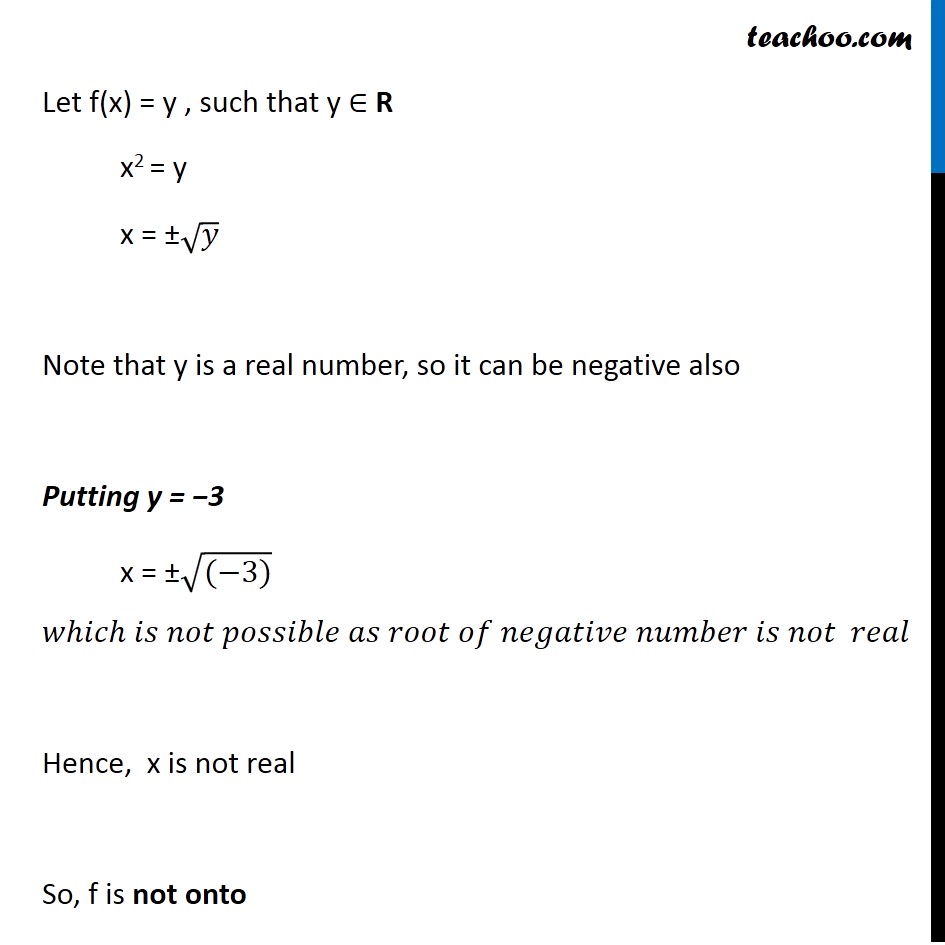
Ex 12, 10 Let A = R − {3} and B = R − {1} Consider the function f A → B defined by f (x) = ((x − 2)/(x − 3)) Is f oneone and onto?Recall the definition of an injective function A function is injective if, whenever $f(x)=f(y)$, it must follow that $x=y$ Now, suppose $f(x)=2x1$ is equal to $f(y)=2y1$, then $$2x1=2y1 \Rightarrow x=y,$$ so $f$ is injective $\textbf{$f$ is surjective}$ Stack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange
Click here👆to get an answer to your question ️ Let A = R {3} and B = R {1} consider the function f A → B defined by f(x) = (x 2/x 3) Show that f is one one and onto and hence find f^1Given that f(x) = x/(1 x^{2}) Taking the derivative of f(x) we get f'(x) = (1 x^{2} 2x^{2})/ (1x^{2})^{2}= (1x^{2})/(1x^{2})^{2} = since for all real x, 1Is f(x) = x e^(x^2) injective?
F is surjective if for all y ∈ Y, there exists some x such that f(x) = y In words, f is onto Bijective f is bijective if f is both injective and bijective Onetoone correspondence We say that X and Y are in onetoone correspondence if there exists a bijection f X → Y Inclusion Suppose X ⊆ Y The inclusion function is defined to be ⇒ x 2 x 1 = y 2 y 1 ⇒ (x 2 – y 2) (x – y) = 0 ` ⇒ (x y) (x y) (x – y) = 0 ⇒ (x – y) (x y 1) = 0 ⇒ x – y = 0 x y 1 cannot be zero because x and y are natural numbers ⇒ x = y So, f is oneone Surjectivity When x = 1 x 2 x 1 = 1 1 1 = 3 ⇒ x x 1 ≥ 3, for every x in N ⇒ f(x) willSolution For If f2, \infty)\rightarrow B defined by f(x)=x^24x5 is a bijection, thenB= Connecting you to a tutor in 60 seconds Get answers to your doubts




Show That The Function F R To R Defined By F X X X2 1 For All X Belongs To R Is Neither One One Nor Onto Also If G R To



Let A R 2 B R 1 If F A B Is A Function Defined By F X X 1 X 2 Show That F Is One One Onto Sarthaks Econnect Largest Online Education Community
Show that the function f in A = R {2/3} defined as f(x) = 4x3/6x4 is oneone and onto Hence find f^1 asked in Mathematics by Afreen ( 307k points)Functions can be injections (onetoone functions), surjections (onto functions) or bijections (both onetoone and onto) Informally, an injection has each output mapped to by at most one input, a surjection includes the entire possible range in the output, and a bijection has both conditions be true This concept allows for comparisons between cardinalities of sets, in proofs comparing the Ex 12, 7In each of the following cases, state whether the function is oneone, onto or bijective Justify your answer(ii) f R → R defined by f(x) = 1 x2f(x) = 1 x2Checking oneonef (x1) = 1 (x1)2f (x2) = 1 (x2)2Putting f (x1) = f (x2) 1 (x1)2 = 1 (x2)2 (x1




Ncert Exemplar Solutions For Class 12 Maths Chapter 1 Relations And Functions Avail Free Pdf



2
Thus, f is bijective Option C f (x) = 2x 1 Let f(x 1) = f(x 2) ⇒ 2x 1 1 = 2x 2 1 ⇒ x 1 = x 2 ⇒ f is one one Let f(x) = y, y ∈ Z ⇒ y = 2x 1 ⇒ y 1 = 2x ⇒ x = (y 1)/2 We observe that if we put y=0, then Thus, y = 0 ∈ Z does not have pre image in Z (domain) ⇒ f is not onto Thus, f is not bijective Option D f (x) = x 2 1 let f(x 1) = f(x 2) ⇒ x 1 2 1 = x 2 2 1 ⇒ x 1 2 = x 2 2 ⇒ x 1 = ± x 2(a) Injective if for all x1,x2 ∈X, f(x1) = f(x2) implies x1 = x2 (b) Surjective if for all y∈Y, there is an x∈X such that f(x) = y (c) Bijective if it is injective and surjective Intuitively, a function is injective if different inputs give different outputs The older terminology for "injective" was "onetoone" Thats right As you say $1,1$ both map on $1$ under the function of $x^2$ This means that $f$ cant be injective The definition you had in class pretty much does the same If you have two values like $x=1$ and $y=1$ with property of $f(x) = f(y) = 1$ them $f$ cant be injective because two different values are mapping onto the same value



Show That A Function F R R Given By F X Ax B A B R A 0 Is A Bijective Sarthaks Econnect Largest Online Education Community



Show That The Function F R R Defined By F X X X 2 1 X R Is Neither One One Nor Onto Sarthaks Econnect Largest Online Education Community
Click here👆to get an answer to your question ️ Show that the function fR { 3 }→ R { 1 } given by f(x) = x 2x 3 is a bijectionFor all x 1, x 2 ∈ X In addition, if T , S are bijective jointly separating maps such that T − 1, S − 1 Y → X are jointly separating, then T , S are called jointly bisep arating maps 3Alternatively, f is bijective if it is a onetoone correspondence between those sets, in other words both injective and surjective Example The function f(x) = x2 from the set of positive real numbers to positive real numbers is both injective and surjective Thus it is also bijective




Ex 1 2 10 F X X 2 X 3 Is F One One Onto Class 12



1
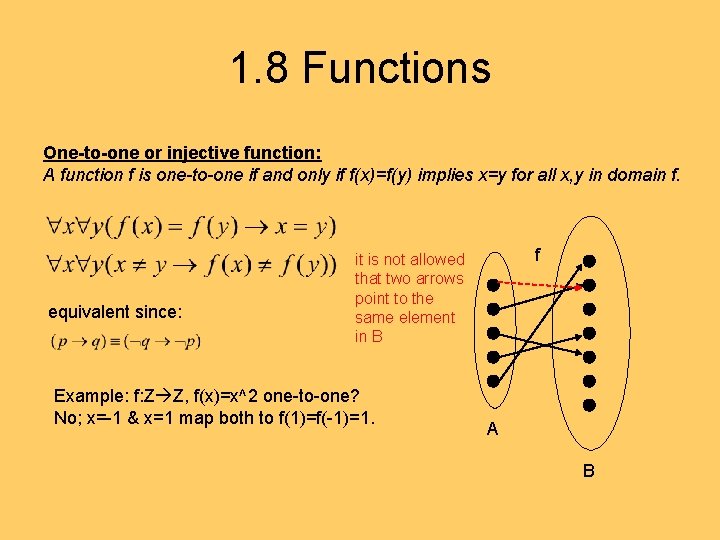
De nition A function f from a set X to a set Y is injective (also called onetoone) if distinct inputs map to distinct outputs, that is, if f(x 1) = f(x 2) implies x 1 = x 2 for any x 1;x 2 2X Example The function f R !R given by f(x) = x2 is not injective as, eg, ( 21) = 12 = 1 In general, you can tell if functions like this are onetoFor f X → Y and g Y → Z functions, prove items 2), 3), 4) from page 123 of lecture notes (a) f and g surjective implies that g f is surjective (b) f and g bijective implies that g f is bijective (c) g f injective implies that f is injective Problem 4Let y be an element in the codomain (Z), such that, f(x)=y ⇒ x2=y ⇒ x=y−2∈Z(Domain) ∴ f is onto ∴ f is bijection f(x)=2x1 one−one test Let x 1
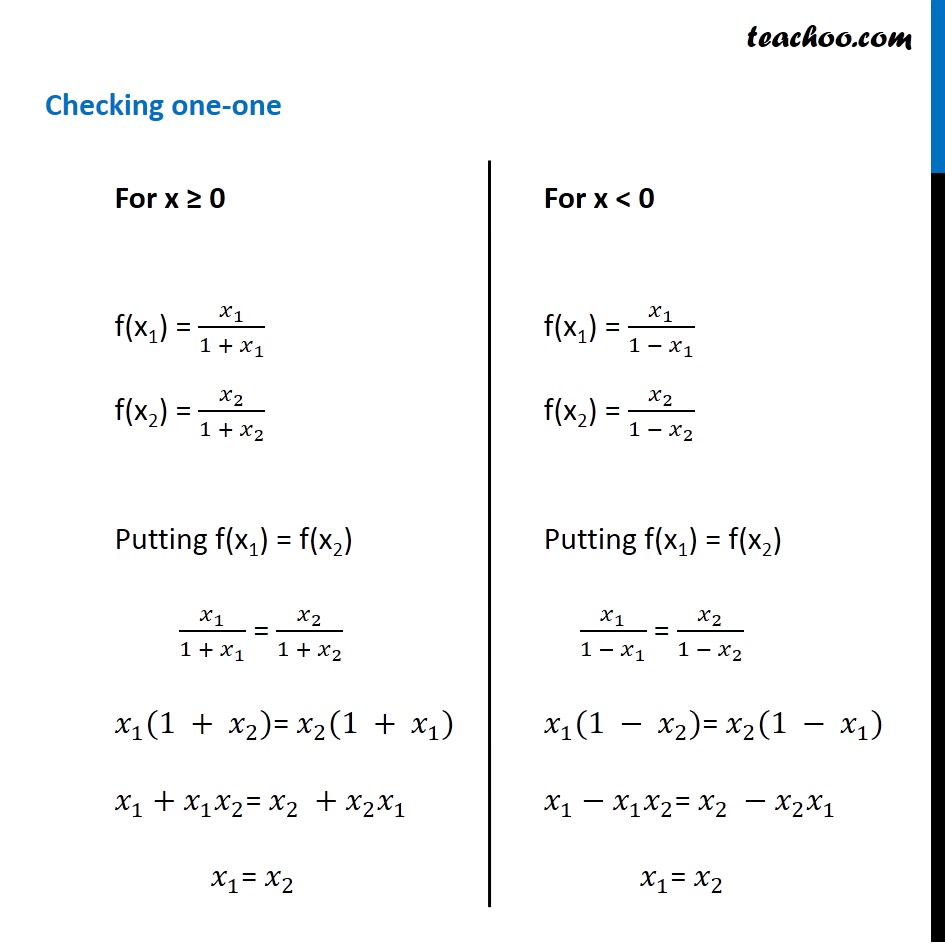



Misc 4 Show F X X 1 X Is One One Onto Miscellaneous




Prove That The Function F Nvecn Defined By F X X 2 X 1 Is One One But Not Onto Youtube
Equivalence Relations and Functions Week 1314 1 Equivalence Relation A relation on a set X is a subset of the Cartesian product X£XWhenever (x;y) 2 R we write xRy, and say that x is related to y by RFor (x;y) 62R,we write x6Ry Deflnition 1 A relation R on a set X is said to be an equivalence relation ifTo understand a bijection, you need to understand 2, simpler concepts Injection and Surjection Let mathf/math be a function with domain A, and codomain B Injection means that every element in A maps to a unique element in B That is to say,Get an answer for 'show that f(x)=x^21 is a bijection x in (1, inf) y in (2,inf)' and find homework help for other Math questions at eNotes




The Function F Rvec 1 2 1 2 Defined As F X X 1 X 2 Is Surjective But Not Injective 2 Neither Injective Not Surjective Invertible 4 Injective But Not Surjective




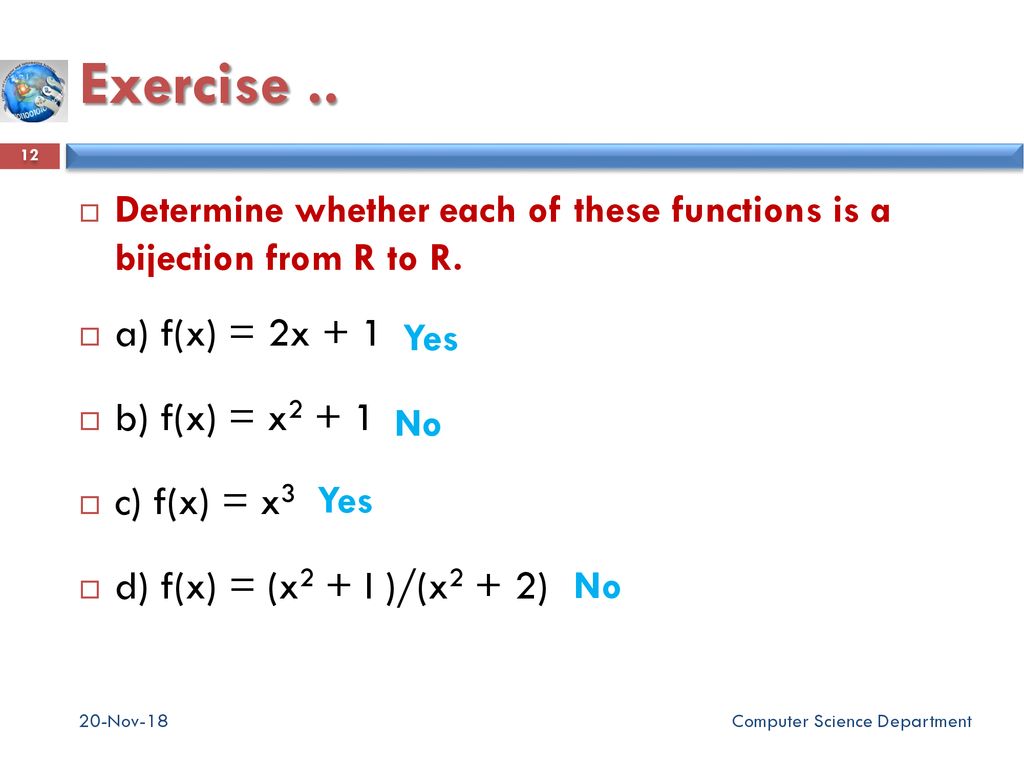
Question 4 Determine Whether Each Of These Functions Chegg Com
The function f X!Y is injective if it satis es the following For every x;x02X, if f(x) = f(x0), then x= x0 In words, fis injective if whenever two inputs xand x0have the same output, it must be the case that xand x0are just two names for the If the function f R – {1, – 1} → A defined by f(x) = x^2/1 x^2, is surjective, then A is equal to Define each of the following (i) injective function (ii) surjective function (iii) bijective function asked Apr 2 in Sets, Relations and Functions by Ekaa (268k points) functions;0 votes 1 answer Are the following



2




Bijection Wikipedia
Let A = R − (2) and B = R − (1) If f A B is a function defined by`"f(x)"=("x"1)/("x"2),` how that f is oneone and onto Hence, find f −1 Ex 12 , 4 Show that the Modulus Function f R R given by f (x) = , is neither oneone nor onto, where is x, if x is positive or 0 and is x, if x is negative f (x) = = , 0 , Misc 5 Show that the function f R R given by f(x) = x3 is injective f(x) = x3 We need to check injective (oneone) f (x1) = (x1)3 f (x2) = (x2)3 Putting f (x1) = f (x2) (x1)3 = (x2)3 x1 = x2 Since if f (x1) = f (x2) , then x1 = x2 It is oneone (injective) Show More Advertisement Miscellaneous Misc 1




12 Determine Whether Each Of The Following Are Chegg Com
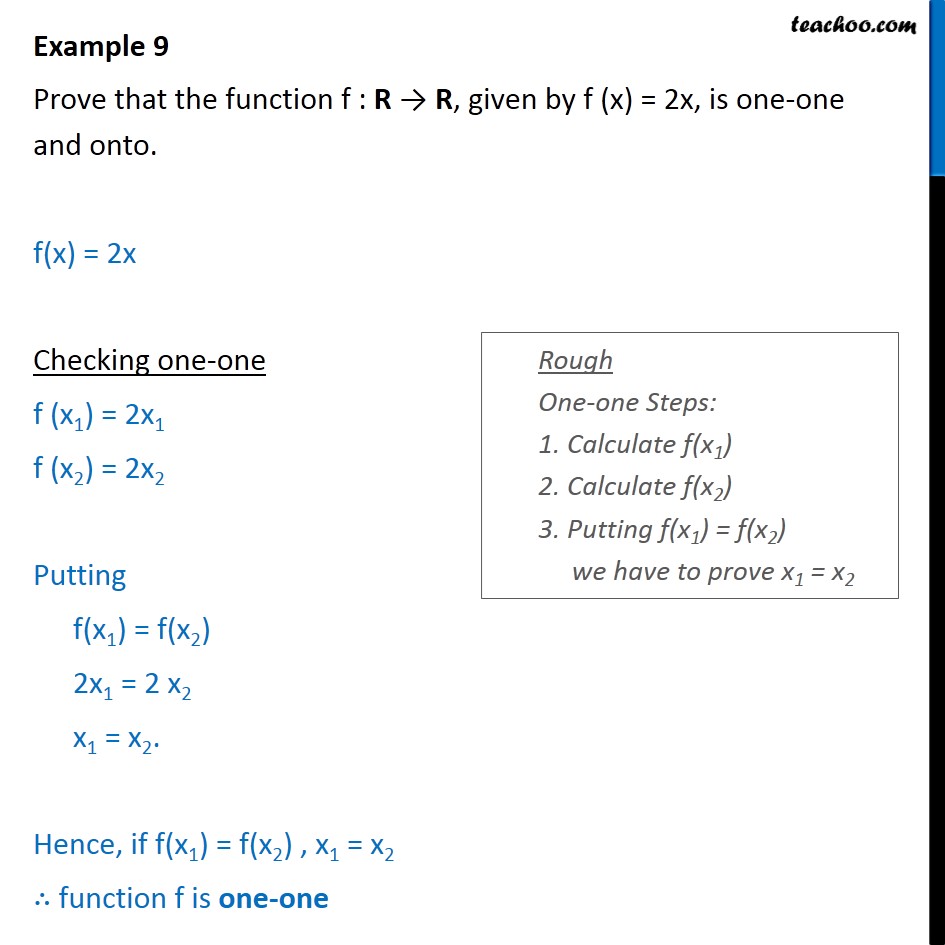



Example 9 Prove That F X 2x Is One One And Onto Chapter 1
Answer ANSWER A=R−{3} B=R−{1} fA→B f(x)= x−3x−2 f(x 1)=f(x 2 )x 1 −3x 1 −2 = x 2 −3 x 2 −2 (x 2 −3)(x 1 −2)=(x 2 −2)(x 1F(x) = 1 e x Theorem 271 If a function is a bijection, then its inverse is also a bijection Proof Let f A!e a bijection and let f 1 B!Abe its inverse To show f 1 is a bijection we must show it is an injection and a surjection Let x 1;x 2 2e such that f 1(x 1) = f 1(x 2) Then by the de nition of the inverse we have x 1 = f(f 1(xExtended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, music




The Function F R 1 2 1 2 Defined As F X X 1 X 2 Is




Q 16 Classify The Following Functions F X Defined In Rrarrr As Injective Surjective Both O Youtube
Math Input NEW Use textbook math notation to enter your math Try itFor the positive real numbers, the given function f(x) = x 2 is both injective and surjective That's why it is also bijective But for all the real numbers R, the same function f(x) = x 2 has the possibilities 2 and 2 So f(2) = 4 and f(2) = 4, which does not satisfy the property of bijectiveF X to 2X, it is obviously to prove X1 = X2 if and only if f (X1)=f (X2), injective or 1–1 Since any elements in form of 2X are from scalar multiplication with 2 by preimage "X", for all X are on domain It is also surjective or onto Therefore, it is bijective which means both injective and surjective 2K views




Chapter 2 Function In Discrete Mathematics



Let A R 3 B R 1 If F A B Be Defined By F X X 2 X 3 X A Studyrankersonline
46 Bijections and Inverse Functions A function f A → B is bijective (or f is a bijection) if each b ∈ B has exactly one preimage Since "at least one'' "at most one'' = "exactly one'', f is a bijection if and only if it is both an injection and a surjection A bijection is also called a onetoone correspondenceNext, let y = 1 There is no x 2Z such that x2 4x 4 = 1 (since x2 4x 4 = (x 2)2) Therefore, h is not surjective 4 Prove that the function f Rf 1g!Rf 1gde ned by f(x) = x 1 x 1 3 is bijective Solution Side work To show that f is surjective, we need to show that for any y 2Rf 1g, we can nd an x such that x 1 x 1 3 = y Take the cubeDefinition 21 Let f X → Y be a function We say f is onto, or surjective, if and only if for any y ∈ Y, there exists some x ∈ X such that y = f(x) Symbolically, f X → Y is surjective ⇐⇒ ∀y ∈ Y,∃x ∈ Xf(x) = y To show that a function is onto when the codomain is a finite set is



How To Prove The Rational Function F X 1 X 2 Is Surjective Onto Using The Definition Youtube




Function Discrete Mathematics Asst Prof Dr Choopan Rattanapoka Ppt Download
//googl/JQ8NysHow to Prove the Rational Function f(x) = 1/(x 2) is Surjective(Onto) using the DefinitionClick here👆to get an answer to your question ️ f R^ → R defined by f(x) = 2^x , x ∈ (0, 1), f(x) = 3^x , x ∈ 1, ∞) isHere, we can see each element of the domain has 2 images So, f is not a function ⇒ If f is not function means it cannot be injective, surjective or bijective




Vatrix Match Type Question Column I 12 F R R Defined By F X X 113 F



Injection Surjection Bijection
Y = 2x 1 Solve for x x = (y 1) /2 Here, y is a real number When we subtract 1 from a real number and the result is divided by 2, again it is a real number For every real number of y, there is a real number x So, range of f(x) is equal to codomain It is onto function Hence it is bijective function (ii) f R > R defined by f (x) = 3 – 4x 2 SolutionThe function f is injective or onetoone if every point in the image comes from exactly one elementinthedomainToshowafunctionisinjectiveprove x 1;x 2 2A and f„x 1



Let F R Rightarrow R Be Given By F X X 2 1 Chegg Com




Let F Be A Function Defined By F X X 1 2 1 Xge1 Statement 1 The Set X F X F 1 X 1 2 Statement 2 F Is A Bijection And F 1 X 1 Sqrt X 1 Xge1




Notes On Topics Of Algebra Notes




Rd Sharma Solutions For Class 12 Maths Exercise 2 1 Chapter 2 Function Get Free Pdf



Show That The Function F R X R 1 R 1 Defined By F X X 1 X X R Is One One And Onto Function Hence Find F 1 X
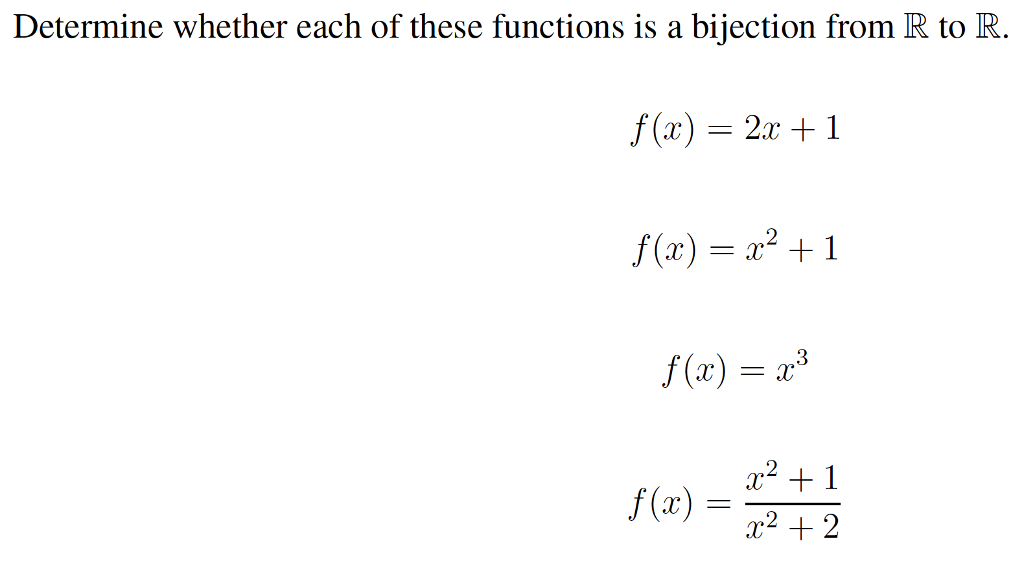



Determine Whether Each Of These Functions Is A Chegg Com



Surjective Onto And Injective One To One Functions Video Khan Academy



Bijection How To Prove W 9 Step By Step Examples



What Is The Inverse Of F X X 2 2x 2 Quora



Example 10 Show F 1 F 2 1 And F X X 1 Is Onto




Let F X X Be Such That F F X X For All X X And X R Then



Cs100 Discrete Structures Ppt Download




1 For Each Of The Following Functions Determine Chegg Com



Q Tbn And9gcri0pfyhep63g05ewsehhkebscejfypkoazculgrhlrfo3lawvg Usqp Cau




The Function F R R F X X 2 Is A Injective But Not Surjective B Surjective But Not Injective C Injective As Well As Surjective D Neither Injective Nor Surjective




Question 13 Determine Whether Each Of These Chegg Com



Web Mat Upc Edu Joaquim Brugues Docs Problems Multivariate Calculus 5 Pdf



Http Library Abes Ac In E Books Engineering mathematics iii module 4 2 Pdf




Classify F R R Defined By F X X X 2 1 As Injection Surjection Or Bijection




B F R R Defined By F X Solution The Function F Chegg Com



Lecture 3 Set Operations Set Functions Recap Set



Math Msu Edu Classes Mth 299 Mth 299 Waiver Example Problems Pdf



1




12 Onto State Whether The Function F Is Bijective Just Math




State Whether The Function Is One One Onto Or Bijective F R R Defined By F X 1 X2 Brainly In




Determine The Function F R R Define By F X X If X Gt 2 5x 2 If X 2 Is A Injection Or Surjection Brainly In
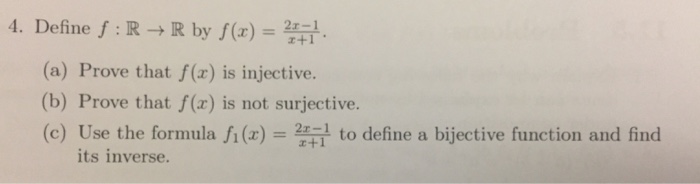



Define F R Rightarrow R By F X 2x 1 X 1 A Chegg Com




Prove That The Function F Nvecn Defined By F X X 2 X 1 Is One One But Not Onto



How To Show That F X X 1 3 2 Is Surjective Discrete Mathematics Math Quora



Injection Surjection Bijection




F X 1 X 2 State Whether Function Is One One Onto Or Bijective



What Is The Inverse Of The Function F X X X 1 Quora




F X 3x 4 Injective And Surjective Mathematics Stack Exchange




Prove That The Function F Nn Defined By F X X2 X 1 Is One One But Not Onto Maths Relations And Functions Meritnation Com




Answered 1 Let F R R Be Defined By F X 2x Bartleby




State Which Of The Following Are One One And Onto Function F R Gtr Defined By F X 2x 1 Youtube




Exercise 1 4 Types Of Functions Problem Questions With Answer Solution Mathematics
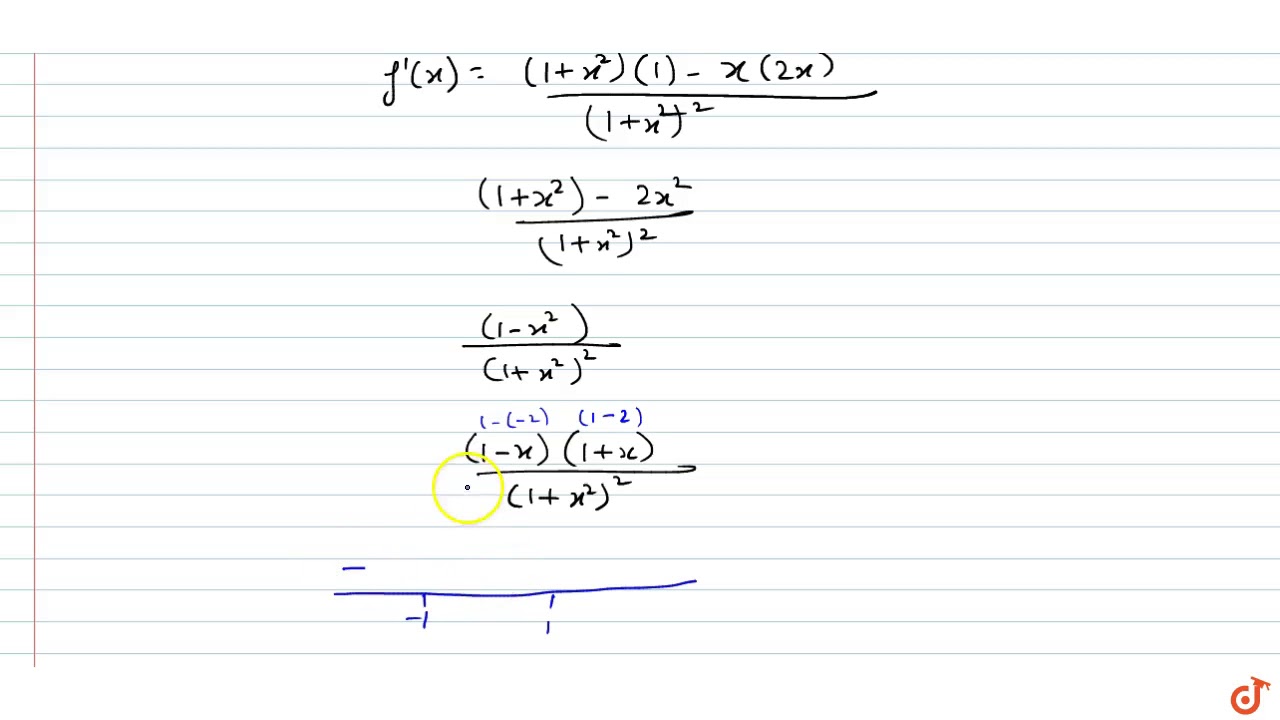



The Function F R Gt 1 2 1 2 Defined As F X X 1 X 2 Is Youtube




If F X Ln X 2 E X 2 1 Then Range Of F X Is Mathematics Topperlearning Com Dbrizw22



Ex 1 2 10 F X X 2 X 3 Is F One One Onto Class 12




Let A X Xepsilonr F Is Defined From Ararrr As F X 2x X 1 Then F X Is A Surjective But Nor Injective B Injective But Nor Surjective C Neither Injective Surjective D Injective



Let A Left X 1 X 1 Right And F A A Such That F X X X Then F Is A A Bijection B Injective But Not Surjective C Surjective But Not Injective D Neither Injective




Ex 1 2 2 I Check The Injectivity And Surjectivity Of F N N



Show That The Function F R R Defined By F X X X 2 1 X R Is Neither One One Nor Onto Sarthaks Econnect Largest Online Education Community




Discrete Mathematics Solutions To Homework 12 For Each Of The Following Sets Determine Whether 2 Is An Element Of That Set Pdf Free Download




How To Determine If A Function Is One To One Mathematics Stack Exchange




Ncert Solutions Class 12 Maths Chapter 1 Relations And Functions Toppers Bulletin




Show That The Function F Rvec Given By F X X 3 X Is A Bijection Youtube




Check The Injectivity And Surjectivity Of Ii F Z Z F X X 2




Show That F R R Defined As F X X 3 Is Bijection Maths Relations And Functions Meritnation Com




Check The Injectivity And Surjectivity Of The Following Functions I F N N Given By F X X2 Cbse Class 12 Maths Learn Cbse Forum




Ex 1 2 10 F X X 2 X 3 Is F One One Onto Class 12



If X 2 Is Not A Bijection Why Are X 3 2 X Bijections Quora




F R R Given By F X X Sqrt X 2 Is A Injective B Surjective C Bijective D None Of These




Check The Injectivity And Surjectivity Of The Following Functions I F N N Given By F X X 2 Ii F Z Z Given By F X X 2 Iii F R



Injective Function Wikipedia




Functions Section 2 3 Of Rosen Spring 10




State Whether The Function F R R Defined By F X 1 X 2 Is One One Onto Or Bijective



The Function F R 1 2 1 2 Defined As F X X 1 X 2 Is Jee Mains Mathematics 17




If The Function F R 1 1 To A Definded By F X X 2 1 X 2 Is Surjective Youtube




Ncert Solutions Class 12 Maths Chapter 1 Relations And Functions Toppers Bulletin




Soni Matrix Match Type 28 Match The Functions Given In Column I Correctly With Mappings Given




If The Function F R 1 1 To A Definded By F X X 2 1 X 2 Is Surjective Then A Is Equal To A R 1 B 0 Oo C R 1 0 D R 1 0




If A Function F 2 Infinity B Defined By F X X 2 4x 5 Is A Bijection Then B 0bq55buu Mathematics Topperlearning Com



Example 11 Show F X X2 Is Neither One One Nor Onto Examples




Ex 1 2 2 I Check The Injectivity And Surjectivity Of F N N



Renethajb Files Wordpress Com 19 09 1 Function Dcs Part 3 Discrete Module 1 Cs 1 Pdf



Part B Set Theory What Is A Set A Set Is A Collection Of Objects Can You Give Me Some Examples Ppt Download




Exercise 1 4 Types Of Functions Problem Questions With Answer Solution Mathematics




2nd Puc Maths Question Bank Chapter 1 Relations And Functions Ex 1 2 Kseeb Solutions




Relation And Function Relation It Is Any Association




Surjective Function Wikipedia



Faculty Math Illinois Edu Rdeville Teaching 347 Hw4s Pdf




Bijection Function F X Y X Y 1 2x 2y 2 Mathematics Stack Exchange



The Function F R 1 2 1 2 Define As F X X 1 X 2 Is Sarthaks Econnect Largest Online Education Community




Tutorial 4 Questions Studocu



Www Whitman Edu Mathematics Calculus Late Calculus Late 09 Transcendental Functions 2up Pdf




5 5 Injective And Surjective Functions Dynamic Slides



Search Q One To One Function Graph Tbm Isch
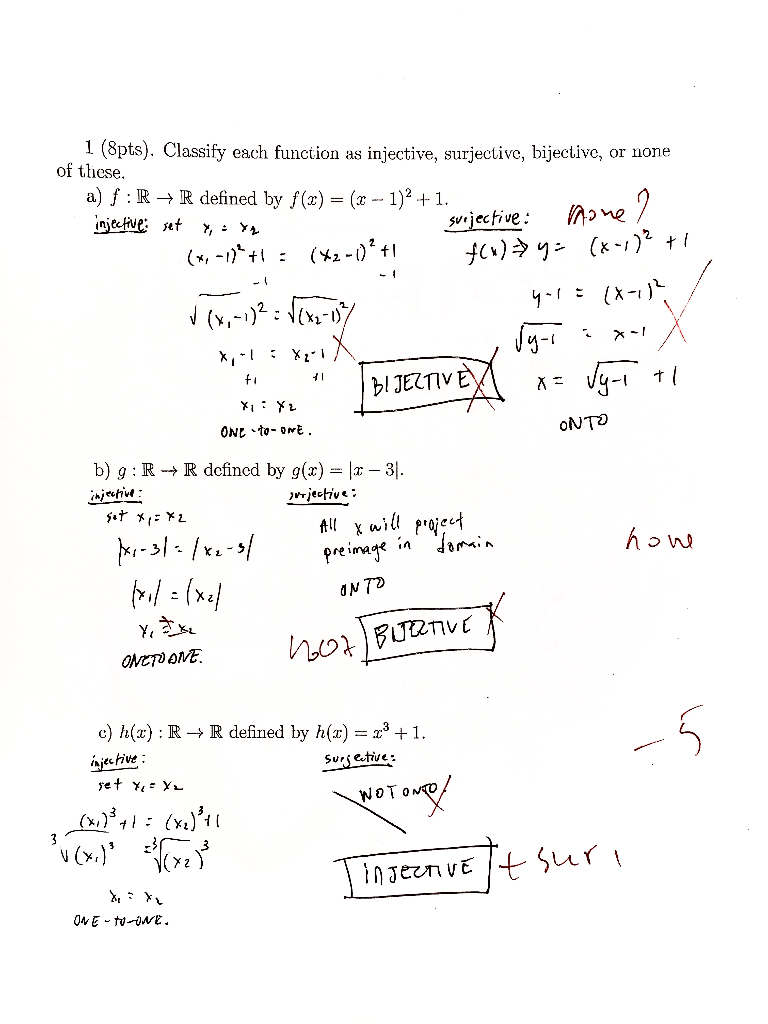



Classify Each Function As Injective Surjective Chegg Com



0 件のコメント:
コメントを投稿